1.5 Monopoly I: Behavior
Contents
Thursday, January 30, 2020 and Thursday, February 6, 2020
Overview
We begin looking at monopoly by discussing how a monopolist (as compared to a price-taking firm) chooses both its output and its price to maximize profits.
Slides
Appendix
Price Elasticity of Demand Refresher
Price elasticity of demand measures how much (in %) quantity demanded changes in response to a (1%) change in price.
\[\begin{align*} \epsilon_{q,p} &= \frac{\% \Delta q}{\% \Delta p}\\ &= \cfrac{\left(\frac{\Delta q}{q}\right)}{\left(\frac{\Delta p}{p}\right)}\\ &= \frac{\Delta q}{\Delta p} \times \frac{p}{q}\\ &= \frac{1}{slope} \times \frac{p}{q}\\ \end{align*}\]
| “Elastic” | “Unit Elastic” | “Inelastic” | |
|---|---|---|---|
| Intuitively: | Large response | Proportionate response | Little response |
| Mathematically: | \(\vert \epsilon_{q_D,p}\vert > 1\) | \(\vert \epsilon_{q_D,p}\vert = 1\) | \(\vert \epsilon_{q_D,p} \vert < 1\) |
| Numerator \(>\) Denominator | Numerator \(=\) Denominator | Numerator \(<\) Denominator | |
| A 1% change in \(p\) | More than 1% change in \(q_D\) | 1% change in \(q_D\) | Less than 1% change in \(q_D\) |
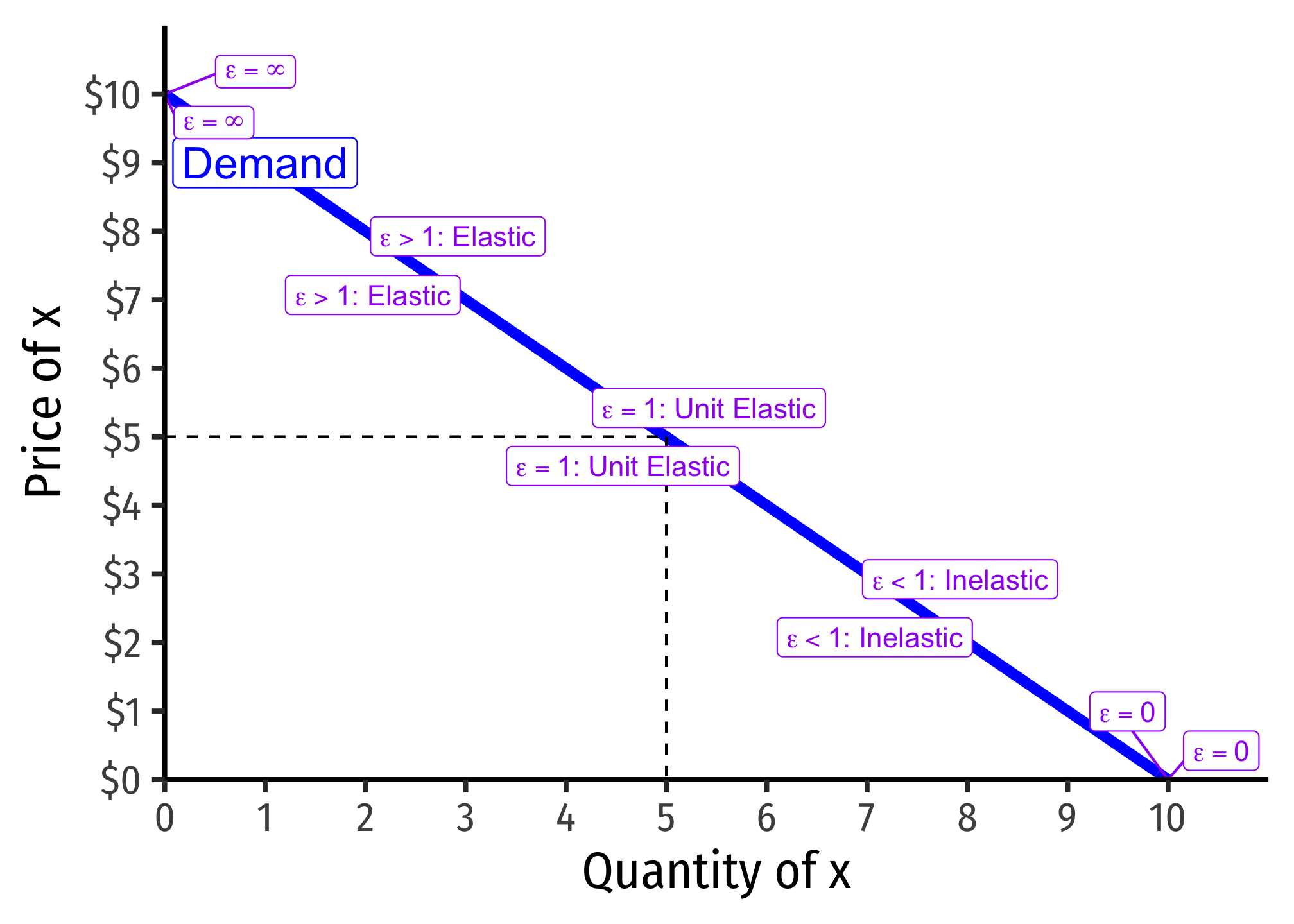
Price elasticity of demand changes along the demand curve:
Determinants of Price Elasticity
What determines how responsive your buying behavior is to a price change?
- More (fewer) substitutes \(\implies\) more (less) elastic
- Larger categories of products (less elastic) vs. specific brand (more elastic)
- Necessities (less elastic) vs. luxuries (more elastic)
- Large (more elastic) vs. small (less elastic) portion of budget
- More (less) time to adjust \(\implies\) more (less) elastic
Derivation of the Lerner Index
Marginal revenue is strongly related to the price elasticity of demand, which is \(E_{D}=\frac{\Delta q}{\Delta p} \times \frac{p}{q}\)I sometimes simplify it as \(E_{D}=\frac{1}{slope} \times \frac{p}{q}\), where “slope” is the slope of the inverse demand curve (graph), since the slope is \(\frac{\Delta p}{\Delta q} = \frac{rise}{run}\).
We derived marginal revenue (in the slides) as: \[MR(q)=p+\frac{\Delta p}{\Delta q}q\]
Firms will always maximize profits where:
\[\begin{align*} MR(q)&=MC(q) && \text{Profit-max output}\\ p+\bigg(\frac{\Delta p}{\Delta q}\bigg)q&=MC(q) && \text{Definition of } MR(q)\\ p+\bigg(\frac{\Delta p}{\Delta q}\bigg) q \times \frac{p}{p}&=\frac{MR(q)}{p} && \text{Multiplying the left by } \frac{p}{p} \text{ (i.e. 1)}\\ p+\underbrace{\bigg(\frac{\Delta p}{\Delta q}\times \frac{q}{p} \bigg)}_{\frac{1}{\epsilon}} \times p &=MC(q) && \text{Rearranging the left}\\ p+\frac{1}{\epsilon} \times p &=MC(q) && \text{Recognize price elasticity } \epsilon=\frac{\Delta q}{\Delta p} \times \frac{p}{q}\\ p &=MC(q) - \frac{1}{\epsilon} p && \text{Subtract }\frac{1}{\epsilon}p \text{ from both sides}\\ p-MC(q) &= -\frac{1}{\epsilon} p && \text{Subtract }MC(q) \text{ from both sides}\\ \frac{p-MC(q)}{p} &= -\frac{1}{\epsilon} && \text{Divide both sides by }p\\ \end{align*}\]
The left side gives us the fraction of price that is markup \(\left(\frac{p-MC(q)}{p}\right)\), and the right side shows this is inversely related to price elasticity of demand.